Wealth & Poverty Review Are We Running Out?
From 1980 to 2020, every one percent increase in population corresponded to a four percent increase in personal resource abundance and an eight percent increase in global resource abundance. Originally published at SubstackIn the movie Avengers: Infinity War Thanos says, “It’s a simple calculus. This universe is finite, its resources, finite. If life is left unchecked, life will cease to exist. It needs correcting.” Thanos is an imaginary comic-book character, so where did Marvel’s writers James Starlin and Mike Friedrich get their inspiration about his ideology of scarcity when they introduced the character in February of 1973?
As anyone who uses Excel knows, modeling is exhilarating and can produce surprising results, especially when using exponential functions. Although he didn’t have Excel, Thomas Malthus birthed the modern practice of employing exponents to create models. In his Essay on the Principle of Population he theorized “Population, when unchecked, increases at a geometrical ratio. Subsistence increases only in an arithmetical ratio. A slight acquaintance with numbers will show the immensity of the first power in comparison with the second.” Like Thanos, Malthus made the condescending implication that mathematics “proved” his theory.
Starlin and Friedrich were creating Thanos in the midst of a period of excessive cultural pessimism. The Vietnam War, the Middle-East oil embargo, and fears of a silent spring combined to produce a dystopian vision of the future. Paul Ehrlich had published The Population Bomb in 1968 and the Club of Rome published Limits to Growth in 1972. Both books obsessed on exponential population growth. From 1950 to 1970 population had been growing at around 1.8 percent a year. While this is technically exponential growth, it is nothing like the “hockey-stick-shaped” growth their cleverly scaled charts depict.
Ehrlich wrote that “Society needs rescaling — we’ve got to reduce the size of the entire human enterprise.” Limits to Growth spends 20 pages exploring the miracle of compound growth. Their primary concern was the “super-exponential rise in world population.” World population in 1972 was around 3.8 billion. It is closer to 7.8 billion today. This indicates a 1.5 percent annual rate of increase, but hardly “super-exponential.” The highest annual growth rate on record was 2.1 percent in 1962. By 2020 it had fallen to 1.04 percent.1 Limits to Growth argued that only way to avoid decline or collapse was to have zero population growth by 1975.
There was an obscure economics professor at the time who read Ehrlich’s book and at first agreed with the scenario but decided he should check the historical facts on resources before accepting the theory. What he discovered was that as population increased, the prices of resources actually decreased. His data suggested that more people made things more abundant. This professor was Julian Simon. He wrote up his findings in Science magazine in the spring of 1980.2 Ehrlich and Simon had a very public debate about the relationship between resources and population. Ehrlich argued that as population increased prices would increase and abundance would decrease. Simon argued the opposite, as population increased prices would decrease and abundance would increase.
Finally in frustration Simon offered to make a bet. Simon let Ehrlich pick any resources and the bet had to be for at least one year. Ehrlich and two of his friends jumped at the offer and selected five metals: copper, chromium, nickel, tin, and tungsten. The bet ran ten years with a basis of one thousand dollars. If inflation-adjusted pries increased, Simon owed Ehrlich the difference. If these prices decreased, Ehrlich owed Simon the difference.
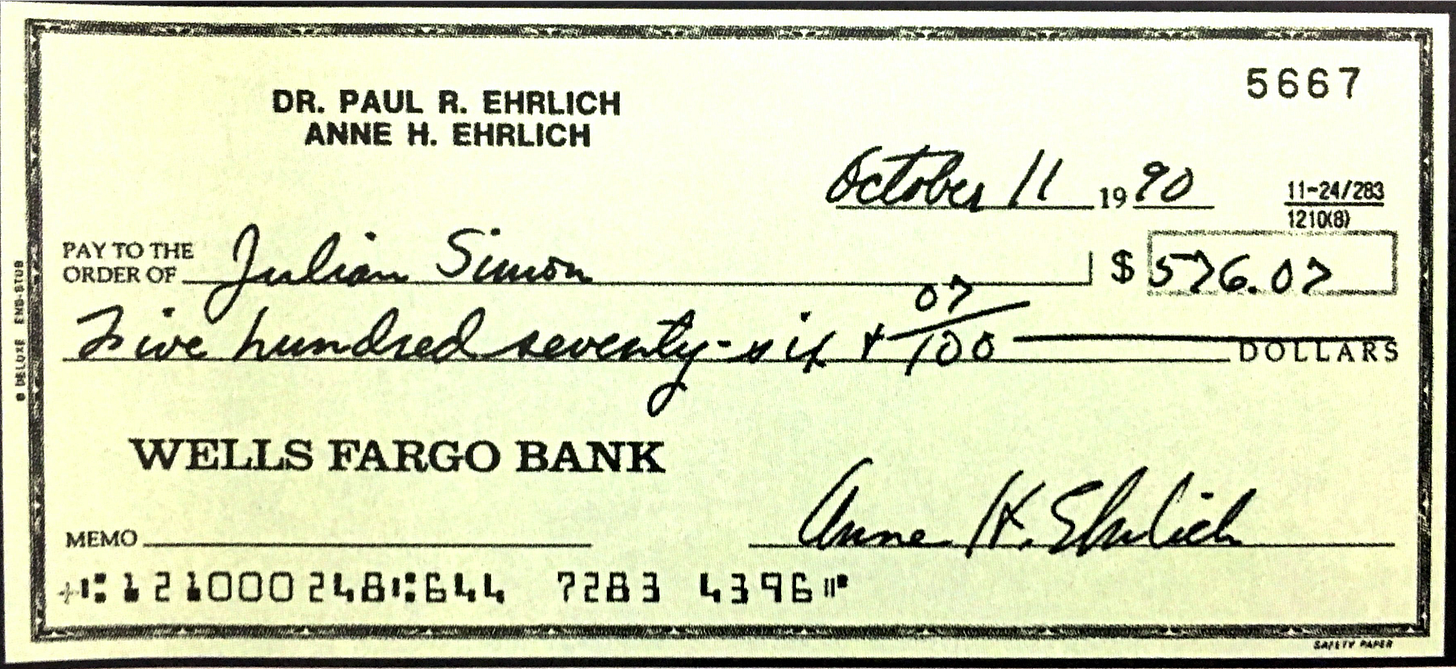
In October of 1990 Paul Ehrlich sent a check to Julian Simon for $576.06. The nominal prices had fallen by 57.6 percent while the inflation-adjusted prices had fallen by 36 percent.
As a followup to this bet, I and Dr. Marian Tupy, senior fellow at the Center for Global Liberty and Prosperity at the Cato Institute, analyzed the relative abundance of 50 basic commodities including energy, food, materials, minerals, and metals from 1980 to 2020.3 We used World Bank, IMF, and Conference Board data and found that the average time price (the time required to earn the money to buy one unit of the commodity) had fallen by 75.2 percent. What this means is that the time required to earn the money to buy one unit in 1980 would get you 4.03 units in 2020. The world experienced a 303 percent increase in personal resource abundance.
During this same 40-year period, global population increased 75.8 percent from 4.434 billion to 7.795 billion. Every one percent increase in population corresponded to a one percent decrease in time prices.
Personal resource abundance increased by 303 percent while global population increased by 75.8 percent. Every one percent increase in population corresponded with a four percent increase in personal resource abundance.
Global resources can be measures as the personal resource abundance multiplied by the size of the population. Since population increased by 75.8 percent, global resources increased by 609 percent. Every one percent increase in population corresponded with an 8.03 percent increase in global resource abundance. More people are making resources much more abundant.
Conclusion
In a sense Thanos was correct, the number of atoms on our earth is pretty much fixed. But economics is not about atoms, economics is about knowledge, and knowledge is not subject to the laws of physics. Thomas Sowell has noted that the difference between our day and the Stone Age is entirely due to the growth of knowledge.4
In 2018 both Paul Romer and William Nordhaus were awarded Nobel prizes for recognizing the true nature of wealth as knowledge and that free people are our ultimate resource, something Julian Simon and Thomas Sowell have said for many years.
Economics is the study of how human beings create value for one another. Value is a function of how intelligently we organize things like atoms, musical notes, words on a page, pictures on a screen, and bits in software. The quantity of things is important, but it’s the value of things that count. And value can change as fast as people can change their minds.

